Solutions
Solutions to Try Its
1. 38 2. [latex]\text{26}\text{.4}[/latex] 3. [latex]\text{328}[/latex] 4. [latex]\text{-280}[/latex] 5. $2,025 6. [latex]\approx 2,000.00[/latex] 7. 9,840 8. $275,513.31 9. The sum is defined. It is geometric. 10. The sum of the infinite series is defined. 11. The sum of the infinite series is defined. 12. 3 13. The series is not geometric. 14. [latex]-\frac{3}{11}[/latex] 15. $92,408.18Solutions to Odd-Numbered Exercises
1. An [latex]n\text{th}[/latex] partial sum is the sum of the first [latex]n[/latex] terms of a sequence. 3. A geometric series is the sum of the terms in a geometric sequence. 5. An annuity is a series of regular equal payments that earn a constant compounded interest. 7. [latex]\sum _{n=0}^{4}5n[/latex] 9. [latex]\sum _{k=1}^{5}4[/latex] 11. [latex]\sum _{k=1}^{20}8k+2[/latex] 13. [latex]{S}_{5}=\frac{5\left(\frac{3}{2}+\frac{7}{2}\right)}{2}[/latex] 15. [latex]{S}_{13}=\frac{13\left(3.2+5.6\right)}{2}[/latex] 17. [latex]\sum _{k=1}^{7}8\cdot {0.5}^{k - 1}[/latex] 19. [latex]{S}_{5}=\frac{9\left(1-{\left(\frac{1}{3}\right)}^{5}\right)}{1-\frac{1}{3}}=\frac{121}{9}\approx 13.44[/latex] 21. [latex]{S}_{11}=\frac{64\left(1-{0.2}^{11}\right)}{1 - 0.2}=\frac{781,249,984}{9,765,625}\approx 80[/latex] 23. The series is defined. [latex]S=\frac{2}{1 - 0.8}[/latex] 25. The series is defined. [latex]S=\frac{-1}{1-\left(-\frac{1}{2}\right)}[/latex] 27.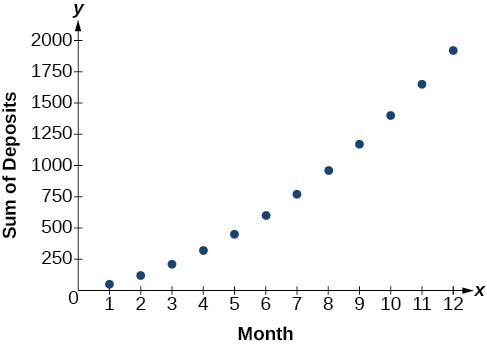
29. Sample answer: The graph of [latex]{S}_{n}[/latex] seems to be approaching 1. This makes sense because [latex]\sum _{k=1}^{\infty }{\left(\frac{1}{2}\right)}^{k}[/latex] is a defined infinite geometric series with [latex]S=\frac{\frac{1}{2}}{1-\left(\frac{1}{2}\right)}=1[/latex].
31. 49
33. 254
35. [latex]{S}_{7}=\frac{147}{2}[/latex]
37. [latex]{S}_{11}=\frac{55}{2}[/latex]
39. [latex]{S}_{7}=5208.4[/latex]
41. [latex]{S}_{10}=-\frac{1023}{256}[/latex]
43. [latex]S=-\frac{4}{3}[/latex]
45. [latex]S=9.2[/latex]
47. $3,705.42
49. $695,823.97
51. [latex]{a}_{k}=30-k[/latex]
53. 9 terms
55. [latex]r=\frac{4}{5}[/latex]
57. $400 per month
59. 420 feet
61. 12 feetLicenses & Attributions
CC licensed content, Specific attribution
- Precalculus. Provided by: OpenStax Authored by: OpenStax College. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
