Putting It Together: Algebra Essentials
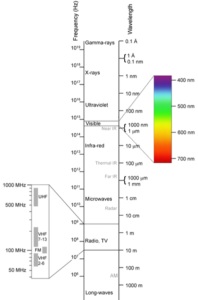 The electromagnetic spectrum with the visible light range enlarged.
The electromagnetic spectrum with the visible light range enlarged.[latex]v=f\lambda[/latex]
where,
[latex]v[/latex] = speed (velocity)
[latex]f[/latex] = frequency
[latex]\lambda[/latex] = wavelength
For example, let’s find the frequency of red light with a wavelength of [latex]7.0\times10^{-7}[/latex]m. First, rearrange to formula to solve for frequency. Then substitute in the given information.[latex]\begin{align}f&=\frac{v}{\lambda} \\[1mm] &=\frac{3.0\times10^8m/s} {7.0\times10^{-7}m}\\[1mm] &=4.0\times10^{14}s^{-1}\end{align}[/latex] Hertz
You can see that wavelength and frequency are inversely related. So blue light, which has a smaller wavelength than red light, will have a greater frequency if traveling at the same speed. So what does it matter if you can calculate the frequency of light? It just so happens that you may be able to predict the future of the universe! If a wave is produced by one object and observed by another, the frequency of the wave changes if either the source of the wave or the observer move relative to the other. This is true for sound waves as well, and you may already be familiar with this phenomenon if you have listened to a siren or train whistle as it approaches and passes you. When it comes to sound, frequency determines pitch so the sound gets higher as it approaches and lower as it moves away. This is known as the Doppler effect. Now back to the universe. Objects in the universe, such as stars, give off light. Suppose you are an astronomer on Earth. If the source of light is moving toward Earth, the frequency of the light will increase. An increase in frequency means that it shifts toward the blue end of the spectrum, and is appropriately known as a blueshift. If the source of light is moving away from Earth, the frequency will decrease and shifts toward the red end of the spectrum. This shift, as you may have guessed, is a redshift. Astronomers have used their understanding of the Doppler effect to make measurements regarding light from distant galaxies. As a result, they have learned that galaxies are moving apart from one another and the universe is expanding. Furthermore, the speed at which an object moves away is proportional to its distance. Because of the tremendous distances in space and the fast speed of light, none of this would be possible without being able to use formulas involving scientific notation.Licenses & Attributions
CC licensed content, Original
- Putting It Together: Algebra Essentials. Authored by: Lumen Learning. License: CC BY: Attribution.
CC licensed content, Shared previously
- Electromagnetic Spectrum. Authored by: transferred by Penubag. License: CC BY-SA: Attribution-ShareAlike.

